top of page

The Forms or elements of the Eternalflower system consists out of similar rings or circles in all its varieties so 1+1 or rather 0+0+0+0+0+0 the ring can be connected at 4,8,3,6,5,10 places in the basics where the 6 connections is the quantum or 2 dimensional level and the rest is multi dimensional. Every ring has a opposite ring except for the core so each core can be split and each structure can fusion into a core. Every point where 2 rings are connected they have possible opposite rings as in figure 8 forming interacting donuts.
We start with the cube it has 3, 4 and 6 dimensions.
The rings are lined out with the 3 dimensions. but when you turn the 4d corners in rings you get the octahedon.

cube

octahedron

Here you see clearly the link between the corners of the cube and the octahedon.

The tetrahedron comes forth out of elong gating the rings of the octahedron in figure 8 configaration. here you see also the vector equalibrium octahedron core forming in the structure.

So the octahedron the cube and the tetrahedron are in the same playing field. Than you would imagine that the 6 dimensions of the cube and octahedon would turn in to the 6 dimensional dodacahedron. But this is not the case if you would turn their 6 dimensions in to rings you get a shape i caled the mentahedron. Of witch you can see it is not a platonic shape and not a stable shape. One could imagine the rings rearanging in the stable dodacahedron shape.

cube 6d

octahedron 6d
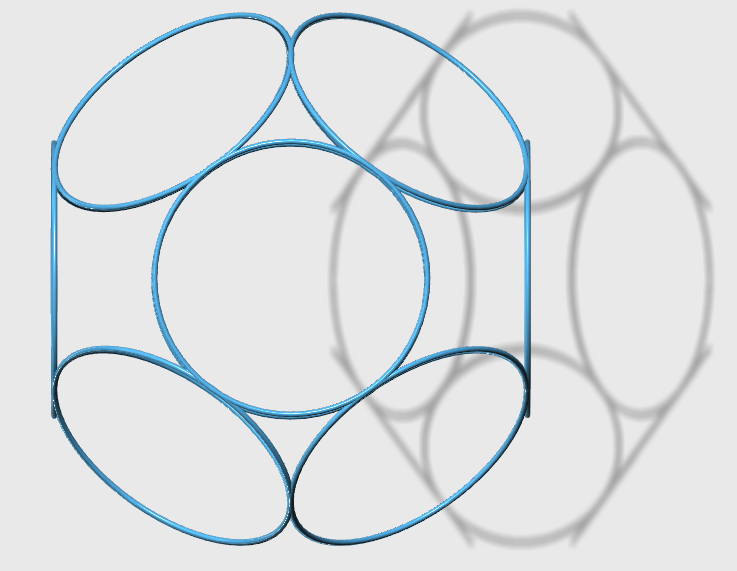
6d mentahedon.
Here you see the 6d dodecahedon when you turn the 10 dimensional corners of the dodecahedron in to rings the isocahedron emerges. So the dodecahedon and isocahedron are conected to etch other like the cube and the octahedron.

dodecahedron 6d

dodecahedron 10d

isocahedron 10d

Here you see the dodecaheron with figure 8 elong gation and forms 5 pointed stars like the octahedon and the tetrahedron.
When one is broken of it forms the element life.

When you take the isocahedron and flip it once around its 15 dimensional axle you will get a shape with all the dimensions and propertys of the cube and octahedron. if you do the same with the dodecahedron the same hapends.






When you flip the 15 dimensions of the isocahedron in to rings you get the maxihedron.



When you flip the maxihedron one time around its 15 dimensional axle it gets all the propertys of the cube.


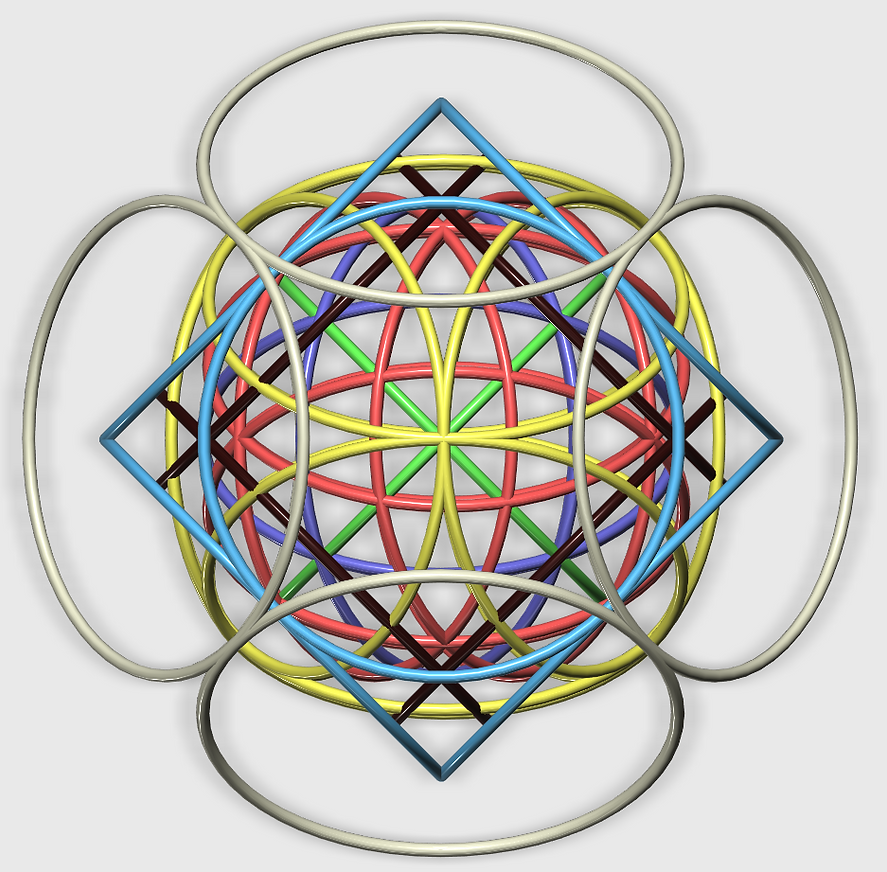
Here you see Interconected.
Green Earth Core
Purple Air Core
Red Double Dark Fire
Yelow Dark Air/Double Fire
Black Dark Earth
Bleu Earth
Grey Air
Yelow and Black Are on the same field.
3D 4D and 6D point of vieuw.



Here you see interconected.
green dodeca core
red Dark Berend
grey berendhedron
yellow dark dodeca
bleu dodecahedron.
6D 10D 15D point of vieuw.



Here you see the both from above togetter showing all the layers until Aether. 9 layers in total.
wass created by putting these lrings lined out between the oposite rings of the dodecahedron.



The ful range of the interconectivenes is far from being discribed. Everytime you twist one element around one axle new posibilitys of twisting in that element apear. Liaving unlimitis amount of variation. In theory in a sudden moment the first ring will reproduce it self.
bottom of page


